gas particles travel in curved paths

Kinetic Molecular Theory of Gases

A Model of Gases as Moving Particles

Yagi Studio/Getty Images

• Thermodynamics
• Physics Laws, Concepts, and Principles
• Quantum Physics
• Important Physicists
• Cosmology & Astrophysics
• Weather & Climate

• M.S., Mathematics Education, Indiana University
• B.A., Physics, Wabash College

The kinetic theory of gases is a scientific model that explains the physical behavior of a gas as the motion of the molecular particles that compose the gas. In this model, the submicroscopic particles (atoms or molecules) that make up the gas are continually moving around in random motion, constantly colliding not only with each other but also with the sides of any container that the gas is within. It is this motion that results in physical properties of the gas such as heat and pressure .

The kinetic theory of gases is also called just the kinetic theory , or the kinetic model,  or the kinetic-molecular model . It can also in many ways be applied to fluids as well as gas. (The example of Brownian motion , discussed below, applies the kinetic theory to fluids.)

History of the Kinetic Theory

The Greek philosopher Lucretius was a proponent of an early form of atomism, though this was largely discarded for several centuries in favor of a physical model of gases built upon the non-atomic work of Aristotle . Without a theory of matter as tiny particles, the kinetic theory did not get developed within this Aristotlean framework.

The work of Daniel Bernoulli presented the kinetic theory to a European audience, with his 1738 publication of Hydrodynamica . At the time, even principles like the conservation of energy had not been established, and so a lot of his approaches were not widely adopted. Over the next century, the kinetic theory became more widely adopted among scientists, as part of a growing trend toward scientists adopting the modern view of matter as composed of atoms.

One of the lynchpins in experimentally confirming the kinetic theory, and atomism is general, was related to Brownian motion. This is the motion of a tiny particle suspended in a liquid, which under a microscope appears to randomly jerk about. In an acclaimed 1905 paper, Albert Einstein explained Brownian motion in terms of random collisions with the particles that composed the liquid. This paper was the result of Einstein's doctoral thesis work, where he created a diffusion formula by applying statistical methods to the problem. A similar result was independently performed by the Polish physicist Marian Smoluchowski, who published his work in 1906. Together, these applications of kinetic theory went a long way to support the idea that liquids and gases (and, likely, also solids) are composed of tiny particles.

Assumptions of the Kinetic Molecular Theory

The kinetic theory involves a number of assumptions that focus on being able to talk about an ideal gas .

• Molecules are treated as point particles. Specifically, one implication of this is that their size is extremely small in comparison to the average distance between particles.
• The number of molecules ( N ) is very large, to the extent that tracking individual particle behaviors is not possible. Instead, statistical methods are applied to analyze the behavior of the system as a whole.
• Each molecule is treated as identical to any other molecule. They are interchangeable in terms of their various properties. This again helps support the idea that individual particles don't need to be kept track of, and that the statistical methods of the theory are sufficient to arrive at conclusions and predictions.
• Molecules are in constant, random motion. They obey Newton's laws of motion .
• Collisions between the particles, and between the particles and walls of a container for the gas, are perfectly elastic collisions .
• Walls of containers of gases are treated as perfectly rigid, do not move, and are infinitely massive (in comparison to the particles).

The result of these assumptions is that you have a gas within a container that moves around randomly within the container. When particles of the gas collide with the side of the container, they bounce off the side of the container in a perfectly elastic collision, which means that if they strike at a 30-degree angle, they'll bounce off at a 30-degree angle. The component of their velocity perpendicular to the side of the container changes direction but retains the same magnitude.

The Ideal Gas Law

The kinetic theory of gases is significant, in that the set of assumptions above lead us to derive the ideal gas law, or ideal gas equation, that relates the pressure ( p ), volume ( V ), and temperature ( T ), in terms of the Boltzmann constant ( k ) and the number of molecules ( N ). The resulting ideal gas equation is:

pV = NkT

• Gases Study Guide
• Topics Typically Covered in Grade 11 Chemistry
• An Introduction to Brownian Motion
• An Overview of Thermodynamics
• Gases - General Properties of Gases
• Pressure Definition, Units, and Examples
• Root Square Mean Velocity Example Problem
• Gas Definition and Examples in Chemistry
• 3 Ways To Increase the Pressure of a Gas
• AP Chemistry Course and Exam Topics
• Fundamental Physical Constants
• Understanding What Fluid Dynamics is
• Biography: Albert Einstein
• The Major Laws of Chemistry
• Chemistry Vocabulary Terms You Should Know
• The Life and Work of Albert Einstein

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

5.5: Thermodynamic States of Ideal Gases

• Last updated
• Save as PDF
• Page ID 27782

• Tom Weideman
• University of California, Davis

State Variables

One of the most important concepts we will use in this course is the idea of a thermodynamic state . There are two key elements to this:

• In thermodynamics, we only deal with equilibrium states . By this we mean that if the physical conditions imposed on the system are not changed, then none of the macroscopically-measurable properties of that state will change. An example in terms of what we have discussed already is the temperature of a sample. We have been assuming that the sample is a uniform temperature throughout its volume. If it were not, then even if we allow no heat to enter or exit the sample (don't change the physical conditions), heat transfers within the sample would still occur, and we would be able to measure temperature changes in various regions of the sample. A sample with differing temperatures occurring in separate regions is not in an equilibrium state.
• For any given equilibrium state, we can completely describe its condition with just a few macroscopically-measurable quantities. So for example, if we have a volume of gas, we can completely define its equilibrium state by measuring its temperature (\(T\)) , volume (\(V\)) , and pressure (\(P\)) . This may not seem to be all that amazing, but the point is that we will see there are many other quantities that can be measured as well ( number of particles (\(N\)) , internal energy (\(U\)) , etc.) that we can compute from our three measured values, making it unnecessary to measure these new quantities separately. That is, the thermodynamic state is completely defined by the measurements of temperature, volume, and pressure, and every other measurable quantity is then uniquely defined by the fact that we know what state the system is in.

All of the measurable quantities like those mentioned above that define a thermodynamic state are called state variables . What is interesting is that we are not required to measure the three specific state variables mentioned earlier in order to completely define the system – we can mix-and-match them! Using the example above, we could measure the number of particles, the pressure, and the internal energy of the gas, and those measurements would be sufficient to compute the other unmeasured state variables like volume and temperature.

Heat and work are also important concepts in thermodynamics, but they are not state variables ! We could have guessed this, since they involve transfers between systems. That is, heat and work are responsible for changing thermodynamic states – they do not serve to define them. As we continue in this subject, we will see how both of these quantities can morph one state into another, thereby changing one or more of the state variables.

Many of the state variables mentioned above require no explanation – we have already discussed temperature, and the meanings of particle number and volume are obvious. We will get to internal energy in due course (as well as other state functions not yet mentioned), but pressure requires a brief introduction. Like temperature, the formal definition of pressure is mathematically complicated, but also like temperature, we can get a sense of what it is by the macroscopic effects it causes. Fluids (and in particular, gases, which we will be studying here) consist of many particles moving in a random fashion. When these particles are confined, they bounce off the walls of the container. The transfer of momentum caused by all these collisions is manifested as a force on the confining wall by the fluid. The amount of force is of course a function of how many particles are hitting the surface at any given moment, which means it is proportional to the area of that surface.

We want to define pressure as a property of the fluid, and not dependent upon the container, so we therefore define pressure of the fluid as the amount of force it exerts on a surface per unit area of that surface. What makes pressure tricky is that it takes into account only the disordered (random) motion of the particles. If the fluid is moving in a macroscopically-measurable way (like a river flowing), then the collisions of the particles that result from this macroscopic motion, while it does account for a force, it does not contribute to the pressure of the fluid. For example, your hand is constantly being pressed in all directions from randomly-moving particles in the air, and the force from these collisions can be used to compute the air pressure. But when you hold your hand out the window of your car as the car moves, the force of the air that you feel on your hand is not air pressure. This is because the motion of the air that contributes to this force is not random – it is ordered because it acts in a single direction.

The units of pressure in SI are: \(\left[P\right] = \dfrac{N}{m^2} \equiv Pa\). The renamed units are called Pascals . There are several other units that are used as well, such as psi (lbs per square inch), and torr (aka millimeters of mercury). We will discuss pressure in greater detail, including the origin of this last odd-sounding unit of measurement in Chapter 7 .

Equations of State

Given that the state of a system is defined by three state variables, with all the other state variables thereby well-defined, there must be some equations that get us from the values of the state variables we measure to the values of the others. These formulas that relate state variables to each other are called equations of state . For example, the pressure of a state could be computed from (i.e. is a function of) volume, the number of particles, and the temperature:

\[P=f\left(V,N,T\right)\]

These relationships between state variables (functions) are not the same in all cases – the relationships depend upon the physical system we are talking about. The type of system we will examine extensively (because it is the simplest one for which we can derive useful information, and because it works very well as an approximation) is that of an ideal gas . Physically, an ideal gas is easy to define: It is a system of particles that are free to move (within the confined space defined by the volume), that never interact with each other. This physical system results in an equation of state (called the ideal gas law ) that relates the four variables mentioned above through the following functional dependence:

\[P=f\left(V,N,T\right) = \dfrac{Nk_BT}{V}\;,\;\;\;\;\; k_B = 1.38\times 10^{-23}\frac{J}{K}\]

where \(k_B\) is called the Boltzmann constant . [ Yes, this is the same Boltzmann mentioned in a previous section on radiative heat transfer. To say that he was a giant in this field would be an understatement. ]

Note that this equation of state involves temperature as an absolute quantity, rather than just the change of temperature, as we saw previously. For this reason, it is necessary to use an absolute scale (kelvins) rather than a comparative scale (celsius) in calculations involving this formula.

A common alternative to using the particle number as a state variable is using the number of moles (\(n\)) . A mole of a gas is simply defined as a specific number of particles of that gas. That "specific number" is known as Avogadro's number : \(N_A \equiv \dfrac{N}{n} = 6.02\times 10^{23}\) .

Writing the ideal gas law in terms of the number of moles is therefore a simple conversion:

\[P = \dfrac{nRT}{V}\;,\;\;\;\;\; R = N_A k_B = 8.31 \frac{J}{mol\;K}\]

The constant \(R\) is known as the gas constant .

Example \(\PageIndex{1}\)

One mole of helium gas is injected into each side of a sliding, airtight lead cylindrical piston of radius \(8.00cm\) that separates two chambers of a sealed cylinder. The outside of the cylinder is insulated everywhere except where noted below. The cylinder and piston have lengths and cross-sectional areas as labeled in the diagram below. The helium in one of the chambers is heated from outside at a rate of \(450\;W\), and the helium in the other chamber expels heat into a cool region. The full system eventually comes to steady-state, so that cooler chamber of gas is losing heat at the same rate as the hotter chamber is receiving it. At this steady state, the pressures of the gases in both chambers are equal to \(9.00\times 10^5 \frac{N}{m^2}\), creating a balance in the forces exerted on the two ends of the piston. The piston therefore remains stationary at an equilibrium point that is a distance \(x\) from the end of the cylinder with the chamber receiving the heat. Find the value of \(x\). The thermal conductivity of lead is \(35.0\frac{W}{m\;K}\).

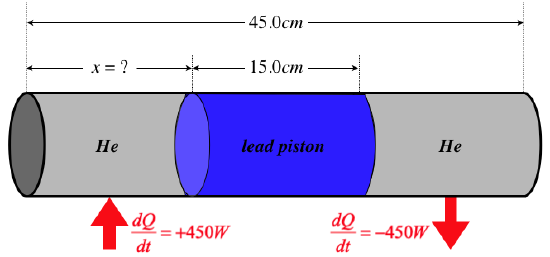
Heat is being conducted from the left chamber to the right one through the lead piston. We are given the rate of heat transfer, the length of the piston, its cross-sectional area, and the thermal conductivity of lead. We can plug all of these into the steady-state heat equation to find the temperature difference:

\[\dfrac{dQ}{dt} = -\dfrac{kA}{L}\Delta T\;\;\; \Rightarrow \;\;\; \Delta T=\dfrac{0.150m}{\left(35.0\frac{W}{m\;K}\right)\pi\left(0.0800m\right)^2} \left(450W\right) = 95.9K \nonumber \]

We are given the pressure of the gases in the two chambers, and the number of moles of gas in each chamber. Using the ideal gas law, we can determine the difference in the volumes of the chambers in terms of the difference in their temperatures:

\[\left. \begin{array}{l} PV_1 = nRT_1 \\ PV_2 = nRT_2 \end{array} \right\} \;\;\;\Rightarrow\;\;\; V_1 - V_2 = \dfrac{nR}{P}\left(T_1-T_2\right) = \dfrac{\left(1mol\right)\left(8.31 \frac{J}{mol\;K}\right)}{9.00\times 10^5 \frac{N}{m^2}}\left(95.9K\right)=885{cm}^3\nonumber\]

Now that we know the difference in the two volumes, we can combine this with the sum of the two volumes to get the volume of the left side:

\[V_1+V_2 = \pi\left(8.00cm\right)^2\left(30.0cm\right)=6030{cm}^3 \;\;\; \Rightarrow \;\;\; V_1 = \frac{1}{2}\left[\left(V_1-V_2\right)+\left(V_1+V_2\right)\right] = \frac{1}{2}\left[\left(885{cm}^3\right)+\left(6030{cm}^3\right)\right] = 3460{cm}^3\nonumber\]

The length of this chamber is its volume divided by its area:

\[x = \dfrac{3460{cm}^3}{\pi\left(8.00cm\right)^2} = 17.2cm\nonumber\]

As with everything else in physics, the idea of an ideal gas is a model . It works pretty well for gases in most real-world circumstances, but it is by no means the only model. Another model treats the gas particles as though they are tiny hard spheres that can bounce off each other. This model may work better in cases of larger molecules and/or higher densities, for example. With a new model comes a new equation of state, and in this case the governing equation is known as the van der Waals equation , which is significantly more complex than the ideal gas law:

\[\left(P+\dfrac{an^2}{V^2}\right)\left(V-nb\right) = nRT \]

The constant \(b\) takes into account the "hard sphere" aspect of the particles. The volume available to the gas is the volume of the vessel minus the volume occupied by the particles themselves. The constant \(a\) accounts for attractive forces (unsurprisingly called van der Waals forces ) between the particles. When the particles attract each other, they don't strike the walls of the container so hard, and the pressure measured by force-per-area on the walls is lower. Notice that taking the limit as \(a,\;b\rightarrow 0\) returns the equation of state back to the ideal gas law. When the particle radii are negligible and interactions forces vanish, then the conditions for an ideal gas are met.

Kinetic Theory of Gases

One of the most impressive aspects of the study of thermodynamics lies in how it is possible to use a simple microscopic model of how a large number (\(~10^{23}\)) of particles in a gas behave to derive some very specific relationships between macroscopically measurable quantities. We see this in action in the following application of the kinetic theory of gases . We will assume a gas is ideal – that the particles do not interact with each other – and that the gas is trapped within a cubical enclosure.

Figure 5.5.1 – Ideal Gas in a Cubical Container

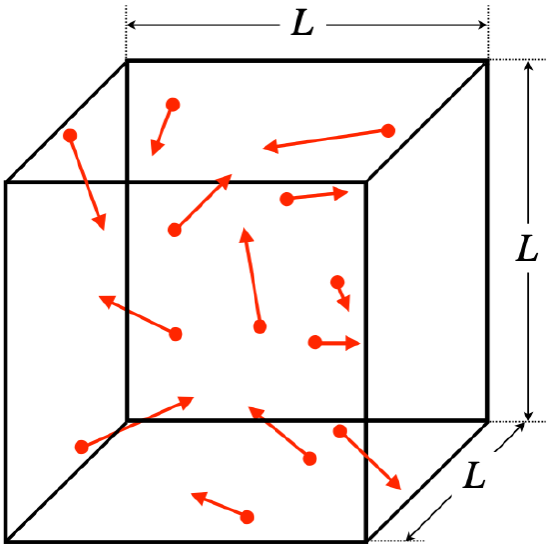
Here are some further assumptions we will make, beyond that of the particles not interacting with each other:

• particles have random positions, speeds, and directions of motion
• no energy lost to the walls (particles collide elastically with walls)
• walls are smooth, so force between particles and wall is perpendicular to wall
• gas is monatomic (individual atoms, not molecules)

We will eventually loosen the last of these constraints, but the others are reasonable and necessary to do the derivation that follows. The assumption that the walls are smooth is not necessary for the final result (nor is the use of a cubical container), but it does make the analysis that follows easier. We will not prove that these simplifying assumptions (smooth surfaces and cubical container) are unnecessary, but at a minimum it should be noted that experimental evidence confirms that the final result works for more general circumstances.

There are literally a trillion-trillion particles in this box, so looking at what they do individually might seem a bit pointless, but in fact we will have the powerful ally of averaging on our side, as you will see. So we forge ahead, looking at the effect of a single particle as it reflects off a wall of the container, hoping to parlay the information we gain into some understanding of pressure, which is manifested as the gas pushing on the walls of the container...

Figure 5.5.2 – Particle Reflection Off Container Wall

A particle that comes into a wall of the container and reflects elastically will exit with the same speed that it has coming in. With the wall being "smooth," there is no force on the particle parallel to the wall, so the component of the particle's velocity parallel to the wall remains unchanged (\(v_y(before)=v_y(after)\)). The elastic collision ensures that the total speed of the particle is unchanged (\(\left|\overrightarrow v_o\right|=\left|\overrightarrow v_f\right|\)). The combination of these two facts means that the component of the particle's velocity in the direction perpendicular to the wall (depicted in the figure above as the \(x\)-axis) is the same before and after the collision with the wall, but in opposite directions.

This particle therefore experiences a force from the wall in the \(+x\)-direction that results in a change of momentum equal to \(2mv_x\). Newton's third law tells us that the wall experiences the same force in the \(-x\)-direction from the particle. If we average this force over a short time that spans the period from shortly before the collision to shortly after, we get:

\[\left(\text{average force on particle over timespan }\Delta t\right) = \dfrac{1}{\Delta t}\int\limits_{t_o}^{t_f}\overrightarrow F dt \]

From the impulse-momentum theorem (a fancy version of Newton's second law), we can replace the time integral of the force with the change in momentum over that timespan:

\[\left(\text{average force on particle over timespan }\Delta t\right) = \dfrac{\Delta \overrightarrow p}{\Delta t} = \dfrac{2mv_x}{\Delta t} \widehat i \]

This particle will strike walls other than the two that are perpendicular to the \(x\)-axis, but for now we will focus only on the component of the particle's motion along the \(x\)-axis. Suppose we wish to know the force that the left wall exerts on this particle averaged over all time. Well, after it bounces off the wall once, it will travel across the box, strike the other wall, and come back again. The \(x\)-component of the particle's velocity won't change at the other wall either, so we know exactly how long it takes the particle to make a round trip – the total distance divided by the speed:

\[\text{time of round trip} = \dfrac{2L}{v_x}\]

With the particle exerting the same force periodically, the average force exerted on this particle over all time is found directly from the last two equations above (we will remove the unit vector from here on, as the direction is clear):

\[\text{average force on particle over all time} = \dfrac{2mv_x}{2L\;/\;v_x} = \frac{1}{L}mv_x^2\]

We now note that this is the average force exerted on just one of the many particles present, and with the particles randomly distributed (one of our assumptions), it is clear that the wall is constantly being pelted with particle collisions. The average force exerted on each particle by the wall is equal to the average force exerted on the wall by each particle (Newton's third law), and since some number of the randomly-distributed particles are constantly hitting the wall, there is no reason to expect that the total force on the wall will fluctuate over time. Therefore the force on the wall by the gas is just equal to the sum of the average forces exerted by all \(N\) particles:

\[\text{force on wall by gas} = F = \frac{1}{L}\sum\limits_N mv_x^2\]

We now define the pressure of the gas as the force it exerts on a surface per unit area of that surface. In the case of the wall of the container, the area is \(L^2\), so we have for the pressure of the gas:

\[P = \dfrac{F}{L^2} = \frac{1}{L^3}\sum\limits_N mv_x^2 = \frac{1}{V}\sum\limits_N mv_x^2\;,\]

where \(V\) is the volume of the cubical container.

Nothing about what we have found here is unique to the walls of the container that are perpendicular to the \(x\)-axis, so we also have:

\[P = \frac{1}{V}\sum\limits_N mv_y^2 = \frac{1}{V} \sum\limits_N mv_z^2\]

Now we can add the last three equations to each other, and to obtain:

\[3P = \frac{1}{V}\sum\limits_N \left(mv_x^2+mv_y^2+mv_z^2\right) = \frac{2}{V}\sum\limits_N \left(\frac{1}{2}mv^2\right)\]

The particles in this gas do not interact, so the only form of energy they possess is kinetic energy. The sum of the kinetic energies of all the particles is the total internal energy of the gas, which gives us a result that no longer includes any reference to individual particles:

\[3P = \frac{2U}{V} \;\;\; \Rightarrow \;\;\; PV = \frac{2}{3} U \]

Given that this is an ideal gas which also satisfies the idea gas law, we can write the internal energy in terms of the temperature:

\[U = \frac{3}{2}nRT \]

We have been saying for awhile that temperature provides a measure of thermal energy, and now we finally have a formula that gives us exactly how these quantities are related. It is truly remarkable that such specific conclusions can be drawn about the macroscopic state of a gas from such simple assumptions about the microscopic behavior of the particles.

Average Particle Speed in a Gas

We can use Equation 5.5.2 and Equation 5.5.14 to draw another conclusion about the particles in this gas:

\[U = \frac{3}{2}Nk_BT\;\;\;\Rightarrow\;\;\; u\equiv \dfrac{U}{N} = \frac{3}{2}k_BT \]

This tells us that the average energy per particle \(u\) is a constant times the temperature of the gas. Keep in mind that the particles’ motions are randomly (but not uniformly) distributed, so while the average particle has this energy, the actual particles have a range of energies.

With the average kinetic energy per particle, we can determine a sort of average velocity of particles in the gas. There are many sorts of averages, and in this case the type we are referring to is called the root-mean-square , or rms velocity, so-named because its calculation involves taking the square root of the mean of the square of the velocity:

\[v_{rms} = \sqrt{\left<v^2\right>} = \sqrt{\frac{2}{m}}\sqrt{\frac{1}{2}m\left<v^2\right>} = \sqrt{\frac{2}{m}}\sqrt{\left<KE\right>} = \sqrt{\frac{2}{m}}\sqrt{\frac{3}{2}k_BT}=\sqrt{\frac{3k_BT}{m}}\]

So the rms speeds of the particles in an ideal gas increase as the square root of the temperature. Also, if the gas is a mixture of particles of different masses, the heavier particles have a lower rms speeds.

The root-mean-square speed of particles in a gas should not be confused with the "usual" definition of "average," where the speeds of all the particles are added together and the sum is divided by the number of particles. To see the difference, consider a "gas" consisting of two particles, one of which is stationary, and the other moving with a speed of \(2v\). These particles have an average speed of \(v\), and an rms speed of \(\sqrt 2 v\). The main reason for preferring to use the rms value of speed is that the rms speed is well-defined by the total energy of the gas and the particle number. This is not true of the standard average speed – many average speeds are possible for the same total energy and particle number.

Video Lecture

• Gases are composed of a large number of particles that behave like hard, spherical objects in a state of constant, random motion.
• These particles move in a straight line until they collide with another particle or the walls of the container.
• These particles are much smaller than the distance between particles. Most of the volume of a gas is therefore empty space.
• There is no force of attraction between gas particles or between the particles and the walls of the container.
• Collisions between gas particles or collisions with the walls of the container are perfectly elastic. None of the energy of a gas particle is lost when it collides with another particle or with the walls of the container.
• The average kinetic energy of a collection of gas particles depends on the temperature of the gas and nothing else.

We know the earth’s atmosphere is a vital element for living. The atmosphere is the layer of invisible gases surrounding the earth. The reason why earth features living beings is because of the atmosphere. The atmosphere covers the entire globe and consists of 99% of nitrogen and oxygen. 1% of the atmosphere is filled with other gases, dust particles and water vapour. In this article, let us know about the last layer of the atmosphere , the exosphere in detail.

Layers of Atmosphere

The atmosphere acts as a barrier to protect the earth from harmful UV (Ultra Violet) rays and lets in moderate sunlight. UV radiations are harmful radiations and are the prime cause of sunburns. There are five layers of the atmosphere; they are:

• Troposphere
• Stratosphere
• Thermosphere

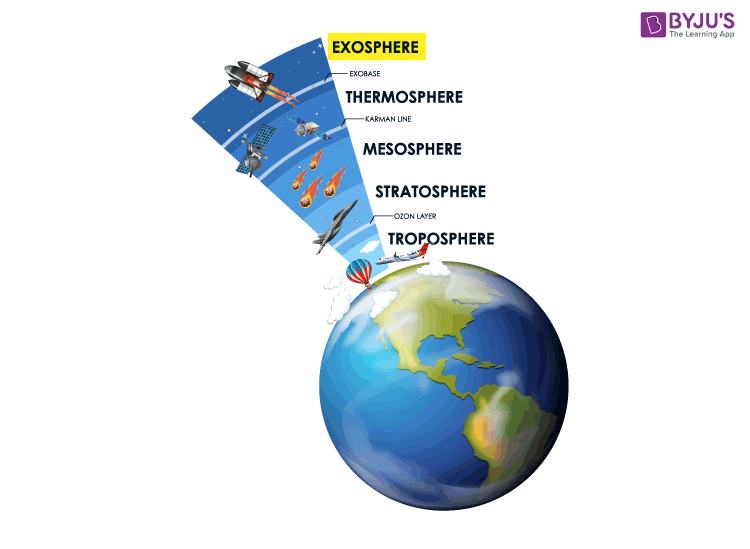
The troposphere is the lowest layer of the earth’s atmosphere. The stratosphere lies above the troposphere and extends up to a height of 50 km. The layer above the stratosphere is known as the mesosphere. This layer extends 85 kilometres from the Earth’s surface. The mesosphere is the coldest layer in the atmosphere, where the temperature goes down as low as -120 degrees Celsius.

The thickest layer of the atmosphere is the thermosphere. Air density is too low in the thermosphere. The lower thermosphere is called the ionosphere, and this ionosphere features electrically charged particles known as ions. The outermost layer of the atmosphere is the exosphere.

Composition and Features of the Exosphere

The outermost layer of the atmosphere and the layer above the thermosphere is the exosphere. Exosphere is derived from the Greek word, where ‘exo’ means outer or external. The region where the exosphere ends, space begins. The Exosphere gradually merges and fades into the vacuum of space.

Air density in this region is extremely thin as hydrogen is the most commonly found gas in the atmosphere. By nature, hydrogen is the lightest element on the earth. The layer below the exosphere witnesses molecules and atoms of atmospheric gases that constantly collide with each other. Along with hydrogen various gases like carbon dioxide, oxygen, and helium are present in the exosphere.

Turbopause is the fluctuating region between the thermosphere and the exosphere. Exobase is the lowest level of the exosphere. The upper boundary of the exosphere is known as geocorona. This boundary is visible to the satellite images of the earth as the blue light that circles the earth.

Facts About Exosphere

• Many satellites, including the notable International Space Station (ISS), orbit within the exosphere or below.
• Exosphere starts at a distance of 311 to 621 miles from the earth’s surface and ends approximately at 6200 miles away from the earth’s surface.
• Exosphere is considered to be the best region to place the satellite
• The pressure in the exosphere is created by solar wind storms that compress it.
• Most gas particles in the exosphere travel in curved paths without colliding with another molecule or atom, eventually arching back into the lower atmosphere due to the gravitational pull.

The video below lets you know about the air and atmosphere

Air and Atmosphere

Stay tuned with BYJU’S for more such interesting articles. Also, register to “BYJU’S – The Learning App” for loads of interactive, engaging Physics-related videos and an unlimited academic assist.

Frequently Asked Questions on Exosphere

1. which is the last layer of the atmosphere.

The exosphere.

2. What is a geocorona?

It is the upper boundary of the exosphere.

3. List the various gases present in the exosphere.

Hydrogen, carbon dioxide, oxygen, and helium.

4. List the five layers of the atmosphere.

5. which is the lowest layer of the earth’s atmosphere.

Troposphere.

Put your understanding of this concept to test by answering a few MCQs. Click Start Quiz to begin!

Select the correct answer and click on the "Finish" button Check your score and explanations at the end of the quiz

Visit BYJU'S for all Physics related queries and study materials

Your result is as below

Request OTP on Voice Call

Leave a Comment Cancel reply

Your Mobile number and Email id will not be published. Required fields are marked *

Post My Comment

• Share Share

Register with BYJU'S & Download Free PDFs

Register with byju's & watch live videos.

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons
• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

2.3: Ideal Gases

• Last updated
• Save as PDF
• Page ID 120463

In an ideal gas, there are no interactions between the particles, hence, the particles do not exert forces on each other. However, particles do experience a force when they collide with the walls of the container. Let us assume that each collision with a wall is elastic. Let us assume that the gas is in a cubic box of length \(a\) and that two of the walls are located at \(x = 0\) and at \(x = a\) . Thus, a particle moving along the \(x\) direction will eventually collide with one of these walls and will exert a force on the wall when it strikes it, which we will denote as \(F_x\) . Since every action has an equal and opposite reaction, the wall exerts a force \(-F_x\) on the particle. According to Newton’s second law, the force \(-F_x\) on the particle in this direction gives rise to an acceleration via

\[-F_x = ma_x = m\dfrac{\Delta v_x}{\Delta t} \label{2.1}\]

Here, \(t\) represents the time interval between collisions with the same wall of the box. In an elastic collision, all that happens to the velocity is that it changes sign. Thus, if \(v_x\) is the velocity in the \(x\) direction before the collision, then \(-v_x\) is the velocity after, and \(\Delta v_x = -v_x - v_x = -2v_x\) , so that

\[-F_x = -2m\dfrac{v_x}{\Delta t} \label{2.2}\]

In order to find \(\Delta t\) , we recall that the particles move at constant speed. Thus, a collision between a particle and, say, the wall at \(x = 0\) will not change the particle’s speed. Before it strikes this wall again, it will proceed to the wall at \(x = a\) first, bounce off that wall, and then return to the wall at \(x = 0\) . The total distance in the \(x\) direction traversed is \(2a\), and since the speed in the \(x\) direction is always \(v_x\), the interval \(\Delta t = \dfrac{2a}{v_x}\). Consequently, the force is

\[-F_x = -\dfrac{mv_x^2}{a} \label{2.3}\]

Thus, the force that the particle exerts on the wall is

\[F_x = \dfrac{mv_x^2}{a} \label{2.4}\]

The mechanical definition of pressure is

\[P = \dfrac{\langle F \rangle}{A} \label{2.5}\]

where \(\langle F \rangle\) is the average force exerted by all \(N\) particles on a wall of the box of area \(A\) . Here \(A = a^2\) . If we use the wall at \(x = 0\) we have been considering, then

\[P = \dfrac{N \langle F_x \rangle}{a^2} \label{2.6}\]

because we have \(N\) particles hitting the wall. Hence,

\[P = \dfrac{N m \langle v_x^2 \rangle}{a^3} \label{2.7}\]

from our study of the Maxwell-Boltzmann distribution, we found that

\[\langle v_x ^2 \rangle = \dfrac{k_B T}{m} \label{2.8}\]

Hence, since \(a^3 = V\),

\[P = \dfrac{N k_B T}{V} = \dfrac{n R T}{V} \label{2.9}\]

which is the ideal gas law.

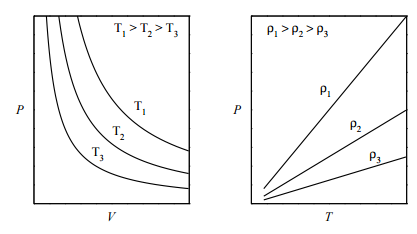
The ideal gas law is an example of an equation of state, which was introduced in Lecture 1. One way to visualize any equation of state is to plot the so-called isotherms , which are graphs of \(P\) vs. \(V\) at fixed values of \(T\) . For the ideal-gas equation of state \(P = nRT/V\) , some of the isotherms are shown in the figure below (left panel): If we plot \(P\) vs. \(T\) at fixed volume (called the isochores ), we obtain the plot in the right panel. What is important to note, here, is that an ideal gas can exist only as a gas. It is not possible for an ideal gas to condense into some kind of “ideal liquid”. In other words, a phase transition from gas to liquid can be modeled only if interparticle interactions are properly accounted for.

Note that the ideal-gas equation of state can be written in the form

\[\dfrac{P V}{n R T} = \dfrac{P \bar{V}}{R T} = \dfrac{P}{\rho R T} = 1 \label{2.10}\]

where \(\bar{V} = V/n\) is called the molar volume. Unlike \(V\) , which increases as the number of moles increases (an example of what is called an extensive quantity in thermodynamics), \( \bar{V}\) does not exhibit this dependence and, therefore, is called intensive . The quantity

\[Z = \dfrac{P V}{n R T} = \dfrac{P \bar{V}}{R T} = \dfrac{P}{\rho R T} \label{2.11}\]

is called the compressibility of the gas. In an ideal gas, if we “compress” the gas by increasing \(P\) , the density \(\rho\) must increase as well so as to keep \(Z =1\) . For a real gas, \(Z\) , therefore, gives us a measure of how much the gas deviates from ideal-gas behavior.

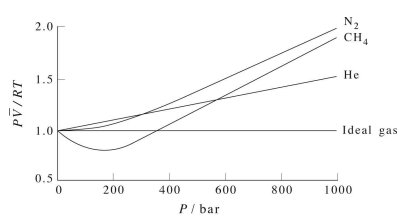
Figure 2.2 shows a plot of \(Z\) vs. \(P\) for several real gases and for an ideal gas. The plot shows that for sufficiently low pressures (hence, low densities), each gas approaches ideal-gas behavior, as expected.

Contributors and Attributions

Mark Tuckerman ( New York University )